Graph Isomorphism
Graphs can look differently but be identical. The only thing that matters are which nodes are connected to which other nodes. If there exists an edge-preserving bijection between two graphs, in other words if there exists a function that maps nodes from one graph onto those of another such that the set of connections for each node remain identical, the two graph are said to be isomorphic.
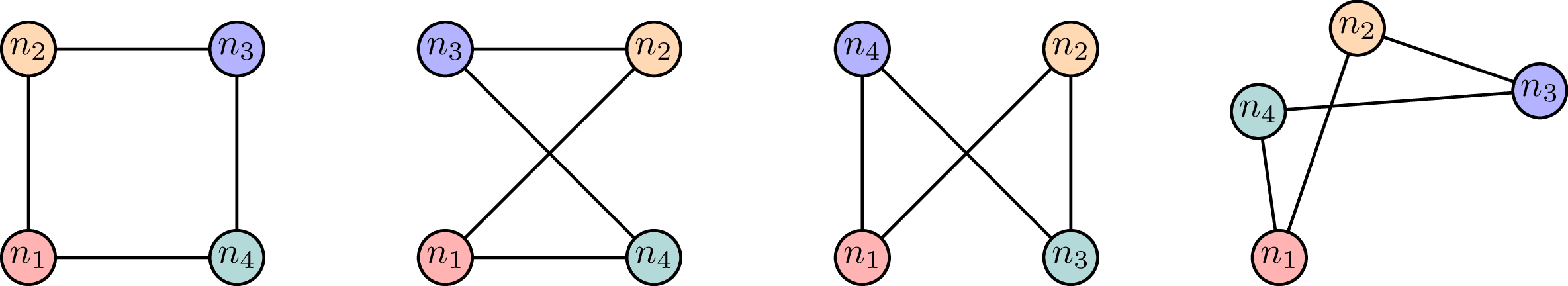
Download
Code
LaTeX
graph-isomorphism.tex (43 lines)
\documentclass[tikz]{standalone}
\begin{document}
\begin{tikzpicture}[
vertex/.style = {circle, draw, inner sep=1pt, fill=white},
vertex1/.style = {vertex, fill=red!30!white},
vertex2/.style = {vertex, fill=orange!30!white},
vertex3/.style = {vertex, fill=blue!30!white},
vertex4/.style = {vertex, fill=teal!30!white},
]
\draw[thick]
(0,0) node[vertex1] (n1^1) {$n_1$}
-- (0,2) node[vertex2] (n2^1) {$n_2$}
-- (2,2) node[vertex3] (n3^1) {$n_3$}
-- (2,0) node[vertex4] (n4^1) {$n_4$} -- cycle;
\begin{scope}[xshift=4cm]
\draw[thick]
(0,0) node[vertex1] (n1^2) {$n_1$}
-- (2,2) node[vertex2] (n2^2) {$n_2$}
-- (0,2) node[vertex3] (n3^2) {$n_3$}
-- (2,0) node[vertex4] (n4^2) {$n_4$} -- cycle;
\end{scope}
\begin{scope}[xshift=8cm]
\draw[thick]
(0,0) node[vertex1] (n1^3) {$n_1$}
-- (2,2) node[vertex2] (n2^3) {$n_2$}
-- (2,0) node[vertex4] (n3^3) {$n_3$}
-- (0,2) node[vertex3] (n4^3) {$n_4$} -- cycle;
\end{scope}
\begin{scope}[xshift=12.5cm]
\draw[thick]
(-0.5,0) node[vertex1] (n1^4) {$n_1$}
-- (0.25,2.2) node[vertex2] (n2^4) {$n_2$}
-- (2,1.6) node[vertex3] (n3^4) {$n_3$}
-- (-0.7,1.4) node[vertex4] (n4^4) {$n_4$} -- cycle;
\end{scope}
\end{tikzpicture}
\end{document}
Typst
graph-isomorphism.typ (68 lines)
#import "@preview/cetz:0.3.2": canvas, draw
#import draw: content, circle, line
#set page(width: auto, height: auto, margin: 8pt)
// Helper function to draw a vertex with a name
#let vertex(pos, label, color, name) = {
content(pos, $n_#label$, frame: "circle", radius: 0.25, fill: color, stroke: black + 0.8pt, name: name, padding: 1pt)
}
#canvas({
// Define colors
let colors = (
rgb("#f9c5c5"), // light red
rgb("#f2ceaa"), // light orange
rgb("#b9d6f2"), // light blue
rgb("#b1e2d8"), // light teal
)
// Draw first graph (square)
let g1-y = 2
vertex((0, g1-y), 1, colors.at(0), "g1n1")
vertex((0, g1-y + 2), 2, colors.at(1), "g1n2")
vertex((2, g1-y + 2), 3, colors.at(2), "g1n3")
vertex((2, g1-y), 4, colors.at(3), "g1n4")
line("g1n1", "g1n2", stroke: black + 0.8pt)
line("g1n2", "g1n3", stroke: black + 0.8pt)
line("g1n3", "g1n4", stroke: black + 0.8pt)
line("g1n4", "g1n1", stroke: black + 0.8pt)
// Draw second graph (trapezoid)
let g2-x = 4
vertex((g2-x, g1-y), 1, colors.at(0), "g2n1")
vertex((g2-x + 2, g1-y + 2), 2, colors.at(1), "g2n2")
vertex((g2-x, g1-y + 2), 3, colors.at(2), "g2n3")
vertex((g2-x + 2, g1-y), 4, colors.at(3), "g2n4")
line("g2n1", "g2n2", stroke: black + 0.8pt)
line("g2n2", "g2n3", stroke: black + 0.8pt)
line("g2n3", "g2n4", stroke: black + 0.8pt)
line("g2n4", "g2n1", stroke: black + 0.8pt)
// Draw third graph (kite)
let g3-x = 8
vertex((g3-x, g1-y), 1, colors.at(0), "g3n1")
vertex((g3-x + 2, g1-y + 2), 2, colors.at(1), "g3n2")
vertex((g3-x + 2, g1-y), 3, colors.at(3), "g3n3")
vertex((g3-x, g1-y + 2), 4, colors.at(2), "g3n4")
line("g3n1", "g3n2", stroke: black + 0.8pt)
line("g3n2", "g3n3", stroke: black + 0.8pt)
line("g3n3", "g3n4", stroke: black + 0.8pt)
line("g3n4", "g3n1", stroke: black + 0.8pt)
// Draw fourth graph (irregular)
let g4-x = 12
vertex((g4-x - 0.5, g1-y), 1, colors.at(0), "g4n1")
vertex((g4-x + 0.25, g1-y + 2.2), 2, colors.at(1), "g4n2")
vertex((g4-x + 2, g1-y + 1.6), 3, colors.at(2), "g4n3")
vertex((g4-x - 0.7, g1-y + 1.4), 4, colors.at(3), "g4n4")
line("g4n1", "g4n2", stroke: black + 0.8pt)
line("g4n2", "g4n3", stroke: black + 0.8pt)
line("g4n3", "g4n4", stroke: black + 0.8pt)
line("g4n4", "g4n1", stroke: black + 0.8pt)
})